Fields
Fields are an imaginary concept where, at every point in space (be it 2d, 3d, or any-d), there exists a value at that point. What this value represents is up to you, as fields are only imaginary abstractions! (yes, including electric fields too).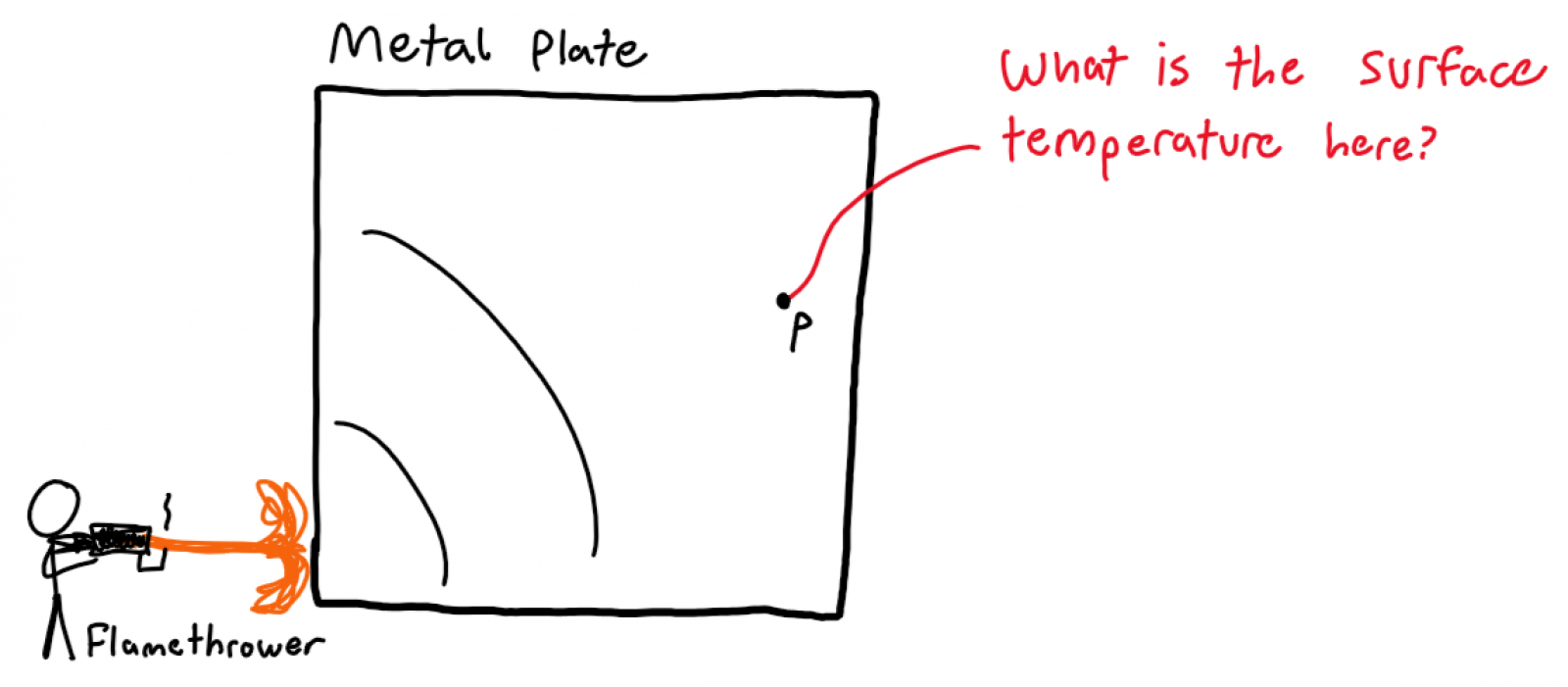
This scalar property is part of a scalar field, as every point on the plate has a temperature you can measure just the same. It's as if you placed an invisible number at every point in space. In this case, a function, exists, which maps the coordinates and to a scalar. 
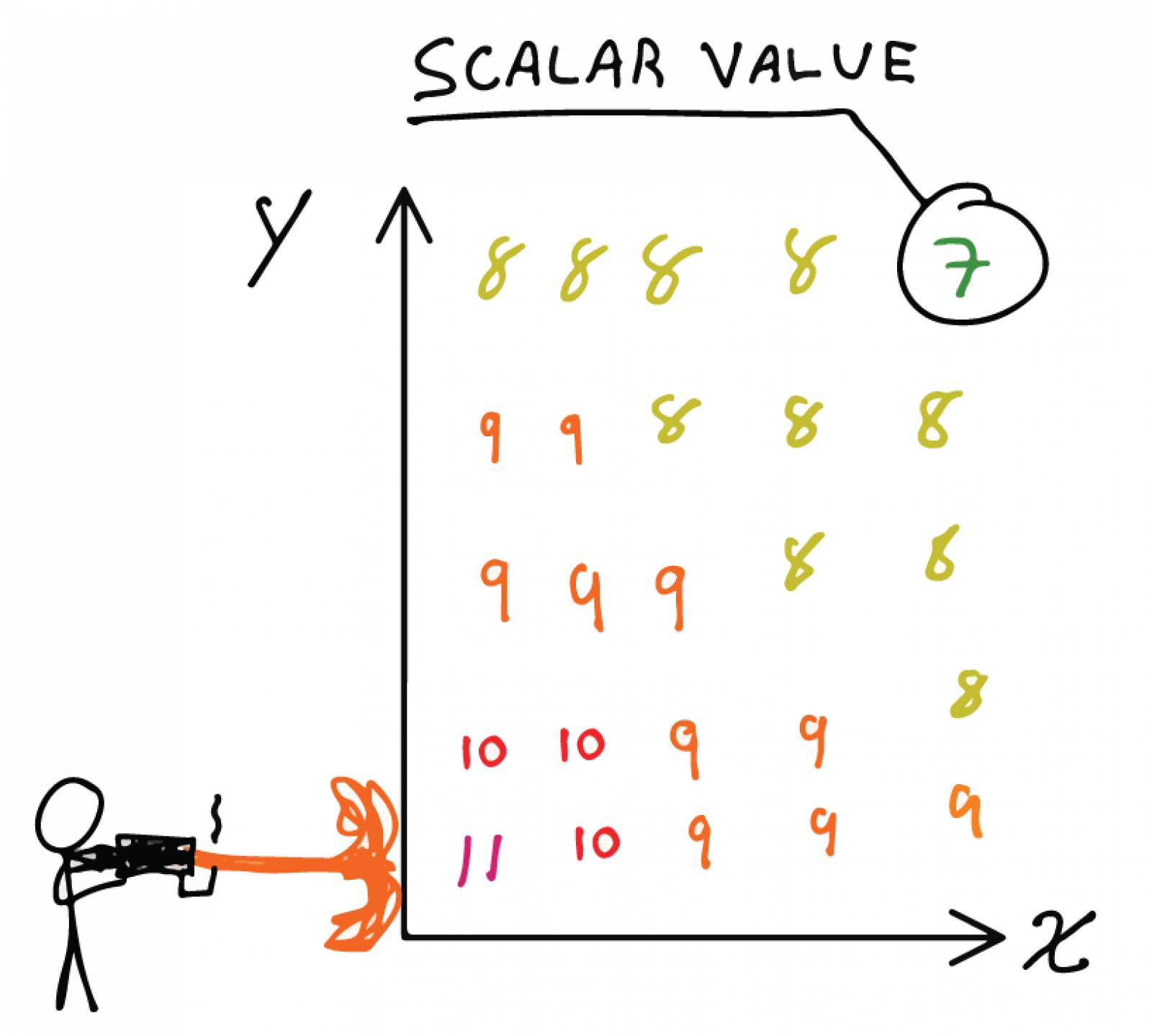Scalar fields are used for measuring simple, one-dimensional quantities such as temperature, pressure, sound pressure level, or color.
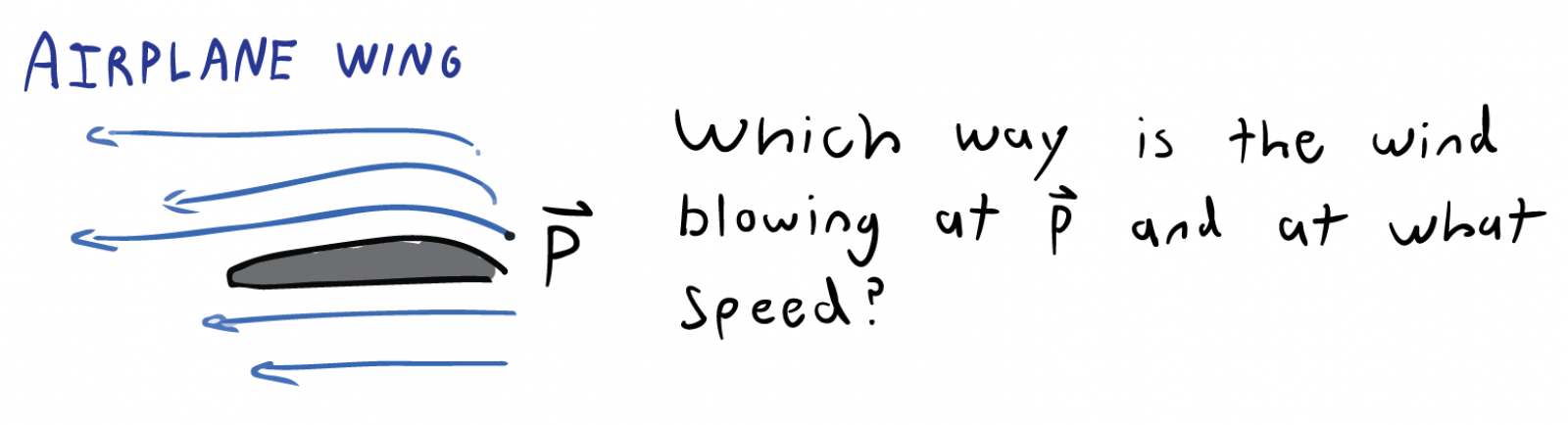This measurement will be a 3d vector, with components in the , , and directions. is our vector field, which maps the coordinates , and to a 3-vector. 
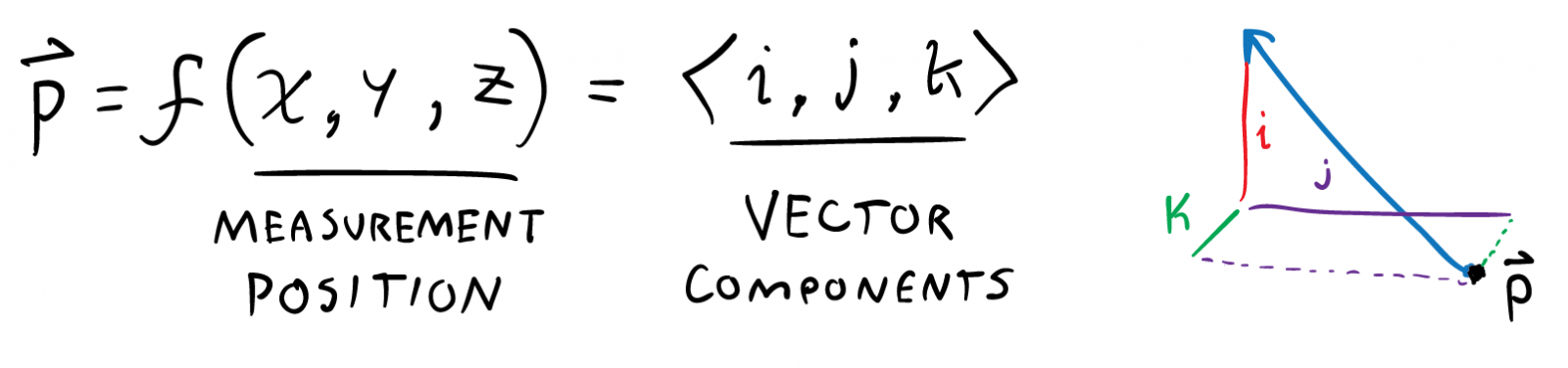This means for every measurement point in 3d space, there exists a 3 dimensional value (a 3-vector). We give the our measurement function a vector and it returns a vector! This is a vector field. 
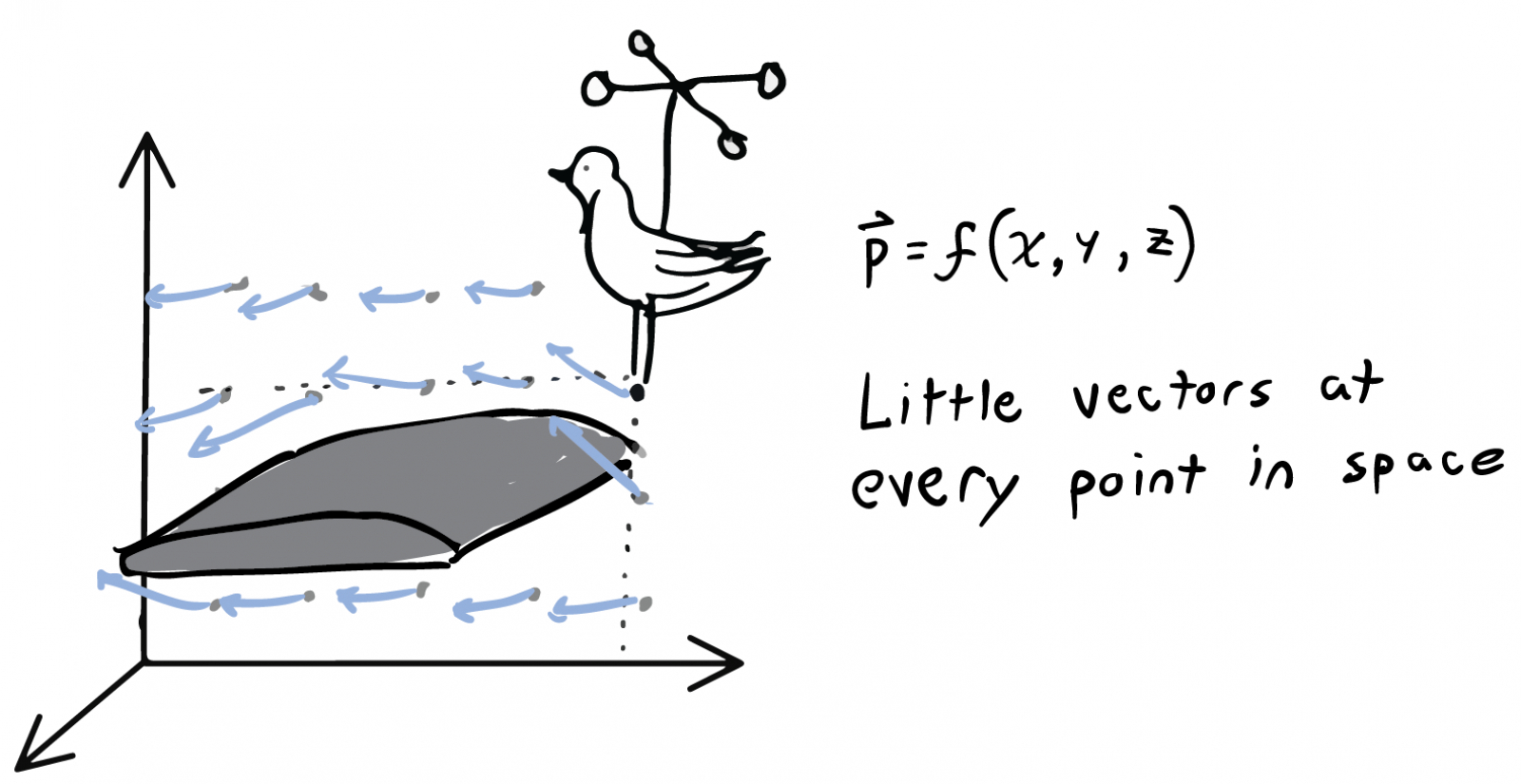Vector fields are incredibly useful for measuring things like flow, electric fields, gravity, acceleration, or anything else where there is force, or directional information of some kind.
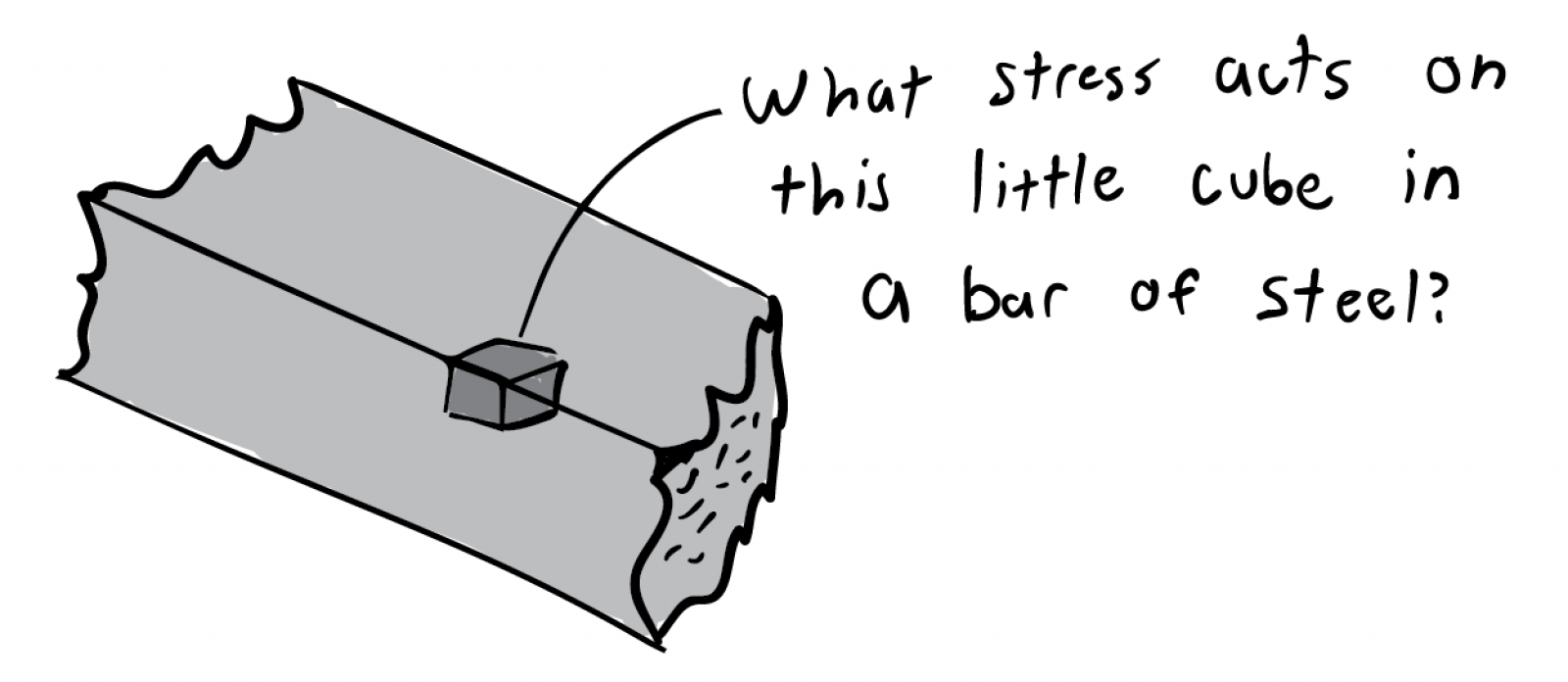At every place in the steel bar, we have a little cube that has stress acting on its planes. 
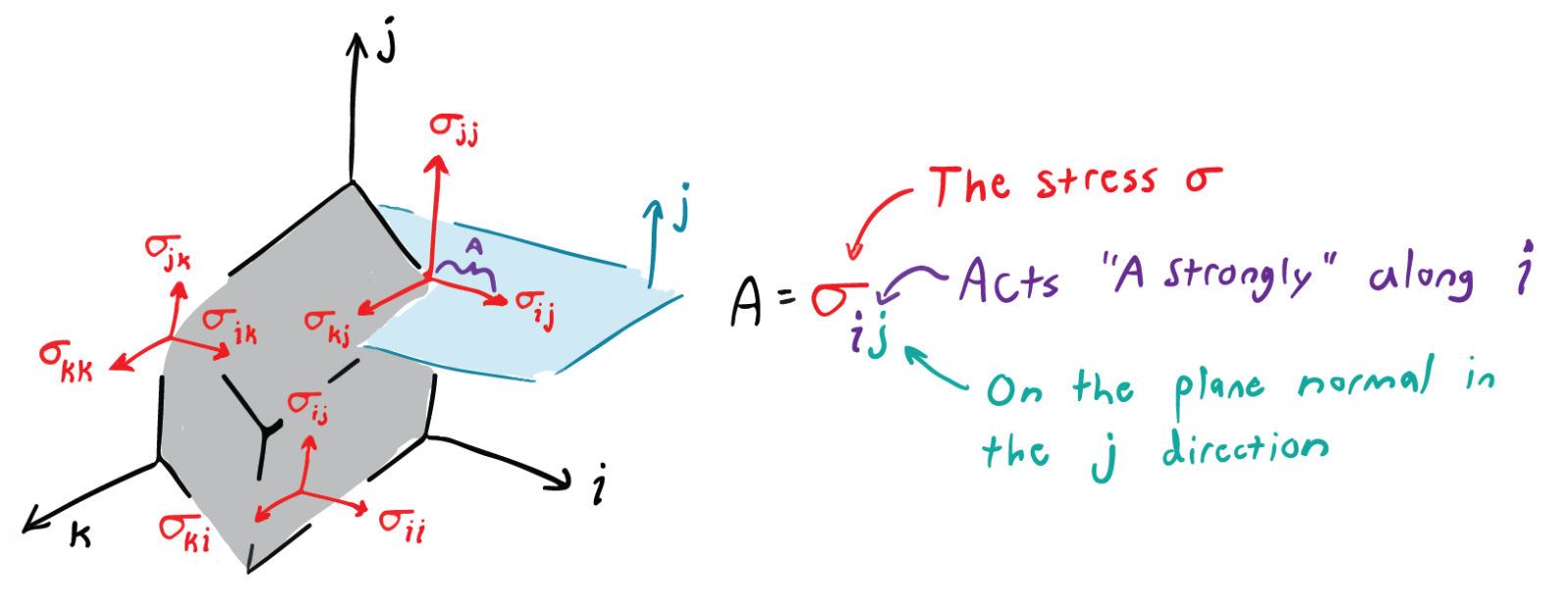We need to know both the direction of the stress, and the direction of the plane it is acting on. To fully describe this stress we need to use a matrix, whcih is a tensor of rank 2.
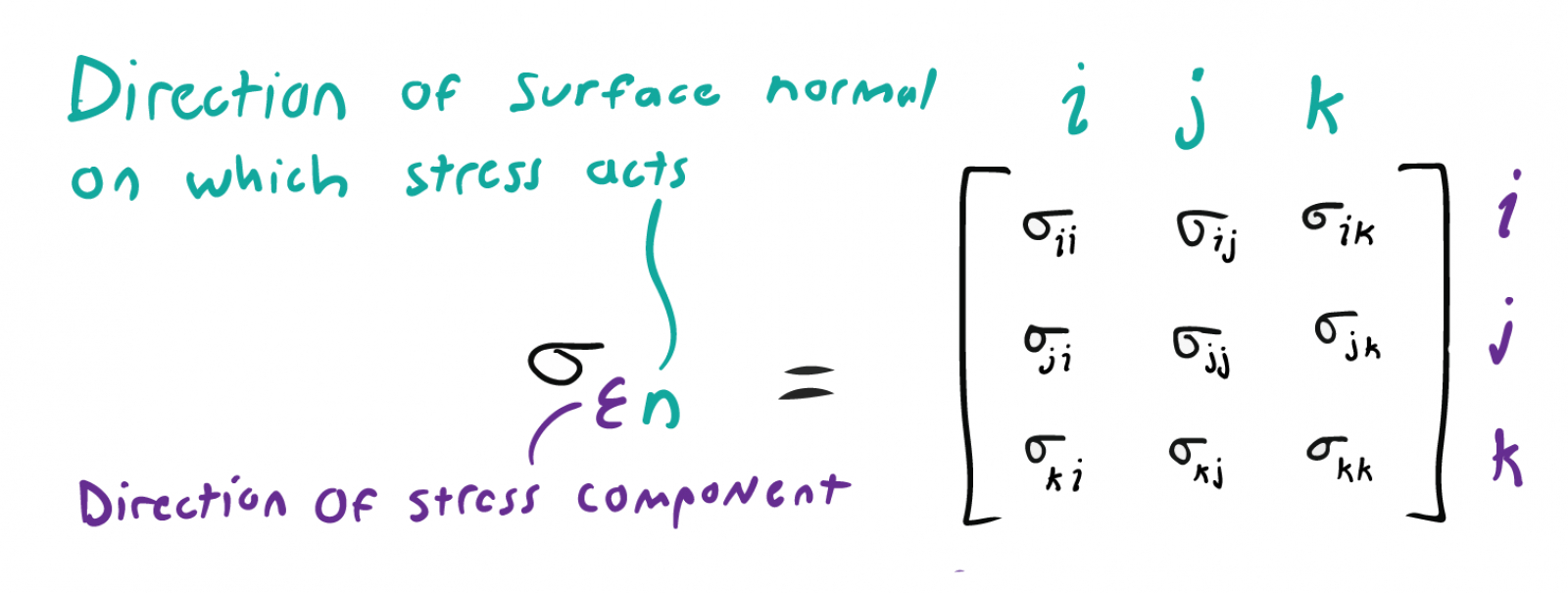Just like a scalar and vector field, here, there is a tensor at every point in space. is our tensor field, which maps the coordinates , and to a rank 2 tensor. 
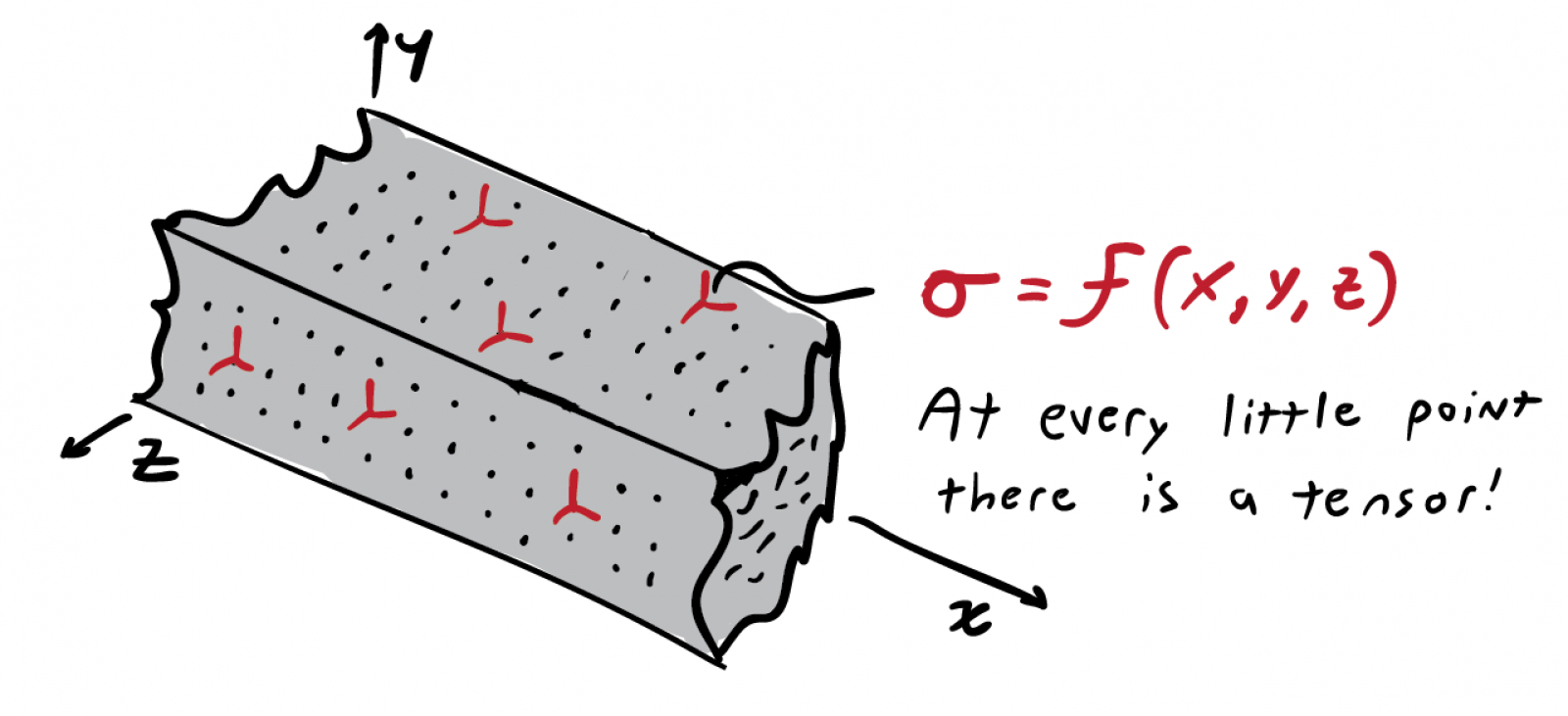In fact, a vector field is just a rank-1 tensor field, and a scalar filed, a rank 0 tensor field!
Scalar Field
Assunme you have some object at a point in space, , and that object has a property you'd like to measure which varies depending on where you are.Vector Field
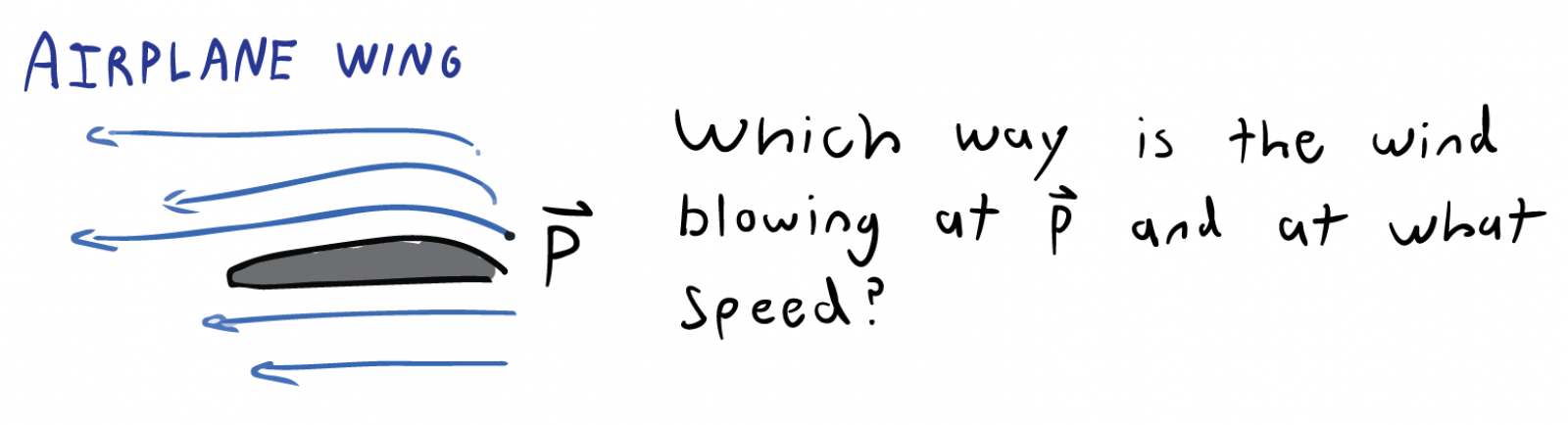
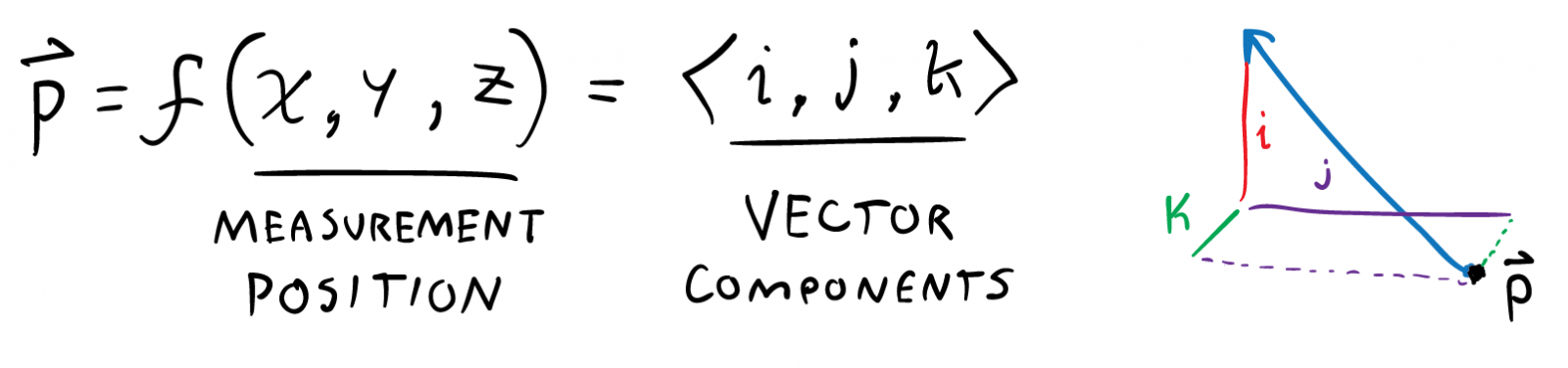
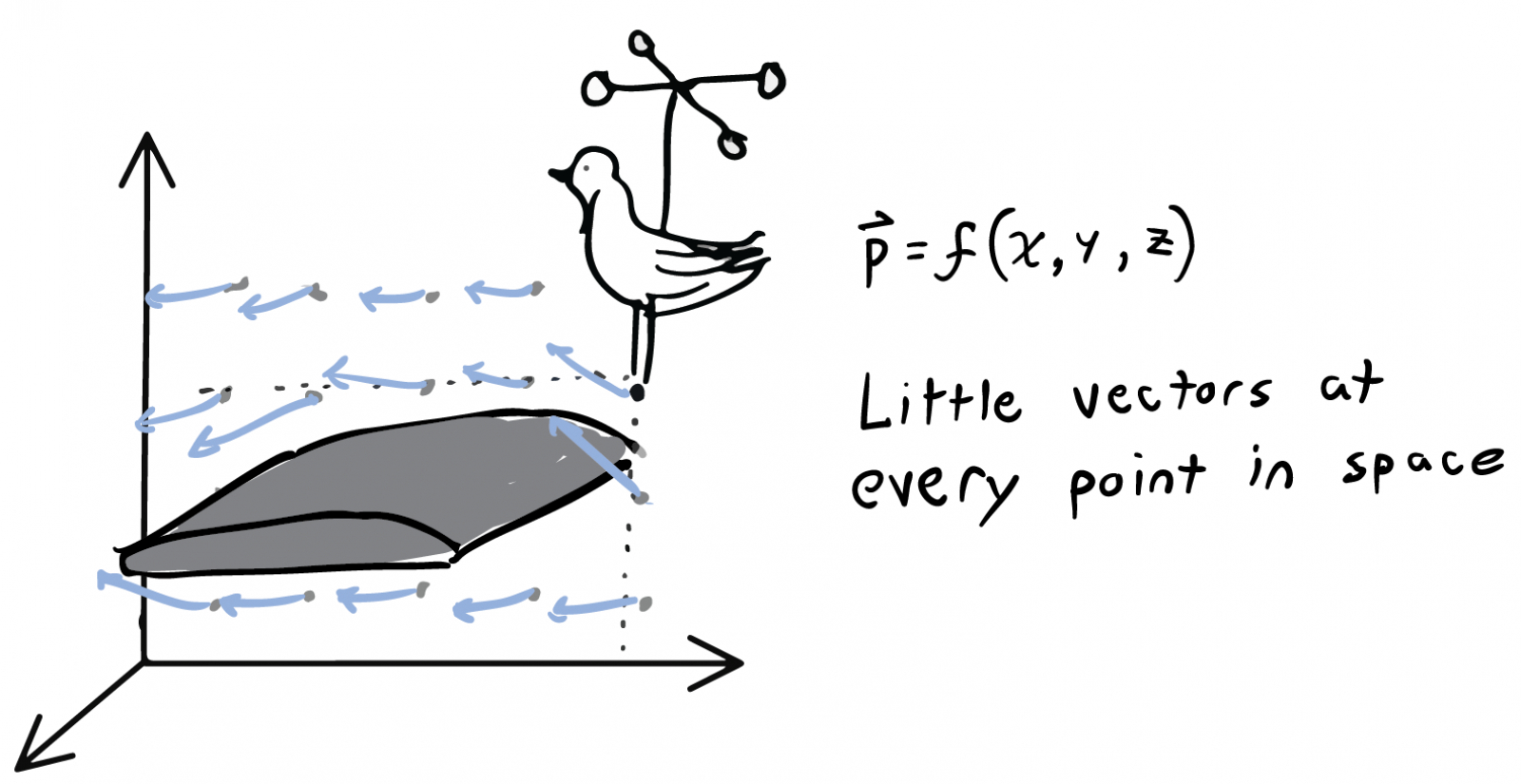
What if what we are trying to measure has a little more dimension to it? Let's say for example, the air flow at a point near an airplane wing.Tensor Fields
What if the property we are trying to measure is very complicated? Too complicated even, for a vector to handle! It's possible that we need to measure something where there is a force at a point, but the force itself also has directions to it! An example of this could be, the stress on a bar of steel.